Product of a Power, Quotient of a Power, and Power of a Power Rules
Once your students have mastered the basics of exponents, moving on to Exponent Rules can be a fun activity for your students. This is because, if they understand exponents, the exponent rules are fairly intuitive.
Product of Exponent Rules
My favorite way to get students to learn the product of powers rule is to put an example problem on the board. For example
23 · 24 = ?
Instead of asking students to solve, especially since they haven’t yet learned to do so. I ask them to think of how they could find the solution since they don’t yet know the rule.
Then I have students write out what 23 is in expanded form. 23 = 2 x 2 x 2
So I write the following on the board
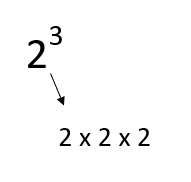
“Great!” I say. “We’re half way there.” I then ask students what 24 represents.
Write next to my 2 x 2 x 2 I write 2 x 2 x 2 x 2
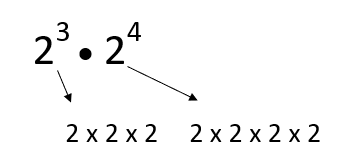
Emphasizing that the first set of twos is for my two to the third power, and my second set is for my two to the fourth power, I ask my students what symbol goes between the two sets of twos.
The goal is to get them to deduce that a multiplication symbol goes between the first and second set of twos, since in the original problem we are multiplying two to the third power times to the fourth power.
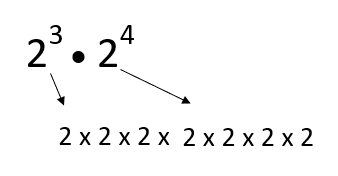
I then have students only focus on the two times itself repeatedly. I ask them how many two’s there are, and we count them together.
“Seven! This is two, times itself, seven times.” I announce to the class. Then I ask, “What’s another way to write two times itself seven times?”
Remembering our exponents, 2 x 2 x 2 x 2 x 2 x 2 = 27
Then, next to the original problem I write the solution. 23 · 24 = 27
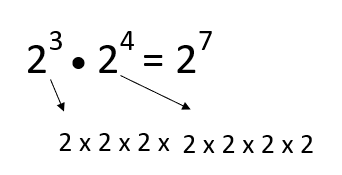
I then ask my students to focus on the exponents. Two to the third power times to the fourth power equals two to the seventh power. “Can anyone think of a rule that might saving us from having to write out all these two times two times twos?”
I wait for my class to realize that three plus four is seven.
So we learned that when two terms with exponents are multiplied by each other (as long as the base is the same) we can find the solution by adding the exponents together.
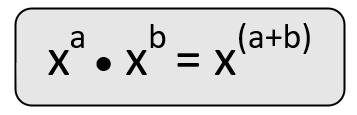
Quotient of Exponent Rules
I love to ask my class the following:
“What is the opposite of multiplication?” (Answer: Division).
“Great.” I explain. “We just did a problem where we multiplied numbers with exponents. What do you think the rule will be when we do the opposite and divide numbers with exponents?”
If they can’t figure it out, I point them towards the rule, xa · xb = x(a+b) and ask if we change the multiplication symbol to a division symbol, what do we think will happen to the addition sign?
After they have correctly surmised the rule, we then put our theory to the test.
I will write out 35 ¸ 32 = ? On the board, and then repeat the process above, this time putting the first term and second term over a division bracket.
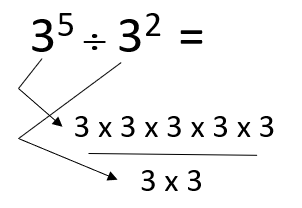
We then get to review our canceling like terms out rule, and I will cross out the two threes on the bottom and two threes on the top of the fraction line.
We then solve, and prove our rule.
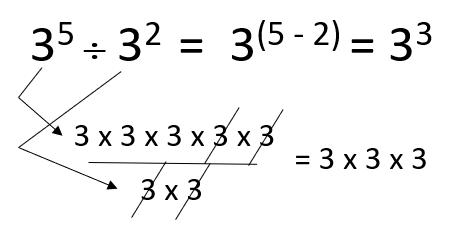
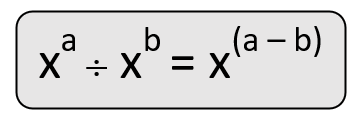
Power of a Power Rule
Finally, We will try to conclude what the rule is when we raise a power to a power.
(24)3 = ?
It is fun to let the students try to guess the rule, but it is sometimes more challenging then we would expect. So we review what an exponent is.
This is two, raised to the fourth power, times itself three times.
24 · 24 · 24
To further illustrate how to solve (in case we’ve forgotten our product of exponents rule that we just learned) I write the following on the board.
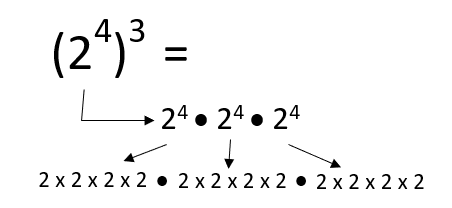
We then count the twos in the bottom row, which of course there are 12. And then we learn our rule.
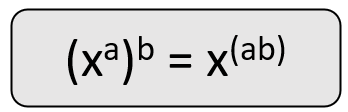
Reteach Worksheets
All of the illustrations given above, which I find to be great tools to help the students learn their exponent rules, are on my Exponent Rules reteach worksheets.
This is a great worksheet to use in class, as you teach students how to do the work. It also works well for independent work, like when a student is absent, or as a response to intervention for a struggling student. Or as my favorite, as a teach or reteach tool inside a learning station.

Making it Permanent
Now that we’ve helped our students learn and understand the exponent rules, we need to help them stick in our students minds. We do this by providing our students with lots of practice, and with immediate feedback.
Here are some great resources to help you do that.
Exponent Rules Worksheets and Activities

Remediate Exponent Skills
If your students are struggling with the Exponent Rules, it might be because they have not yet mastered powers and exponents. It’s hard for them to understand all of this when they’re missing the foundational skills required to do the work.
It’s hard on you to provide remediation for these struggling students without slowing down all of the students who don’t need it. You want to push those students while remediating your struggling ones.
I like to accomplish this through Learning Stations.
I build a learning station for each skill that consists of a reteach and lots of independent practice with immediate feedback. This allows me to reach my struggling students without holding back my ones who have already mastered the prerequisite material.
I have premade learning stations for you so that you can do the same in your classroom. Click the links below
Premade Learning Stations
- Powers and Exponents
- Negative and Zero Exponents
- Exponent Rules: Product, Quotient, and Power of a Power
To preview more premade learning stations, click here